Selective Experiments

To run an experiment with a selective pulse, three parameters must be set - the shape of the pulse, its duration, and its power. On Bruker spectrometers running TopSpin the easiest way to calibrate these parameters is to use Shape Tool. The complete manual for Shape Tool can be found here.

Shape Tool

In TopSpin Shape Tool can be started with the command "stdisp" or by selecting "Spectrometer -> Shape Tool" from the top menu bar. This opens a new window with its own menu bar along the top and changes the options on the menu bar of the main TopSpin window.

Shape

To select a shape to work on click the folder icon on the left of the Shape Tool menu bar, select "shape" from the drop down menu, and pick a shape from the list that appears. The choice of shape depends on the application. The table below gives some suggestions on appropriate shapes for different experiments.

Experiment	Purpose	Shape
1D selective TOCSY, NOESY	180^o ¹H refocusing	Gaus1_180r.1000
2D ¹³C HSQC	adiabatic 180^o ¹³C inverting	Crp60,0.5,20.1
2D band-selective HSQC, HMBC	180^o ¹³C refocusing	G3.256
2D HOBS TOCSY	180^o ¹H refocusing	Reburp.1000
2D HSQC with water flipback	90^o ¹H excitation	Gaus1.1000
2D SO-FAST HMQC	rapid 120^o ¹H excitation	Pc9_4_120.1000
3D triple resonance	180^o ¹³C refocusing	Q3.1000

Duration

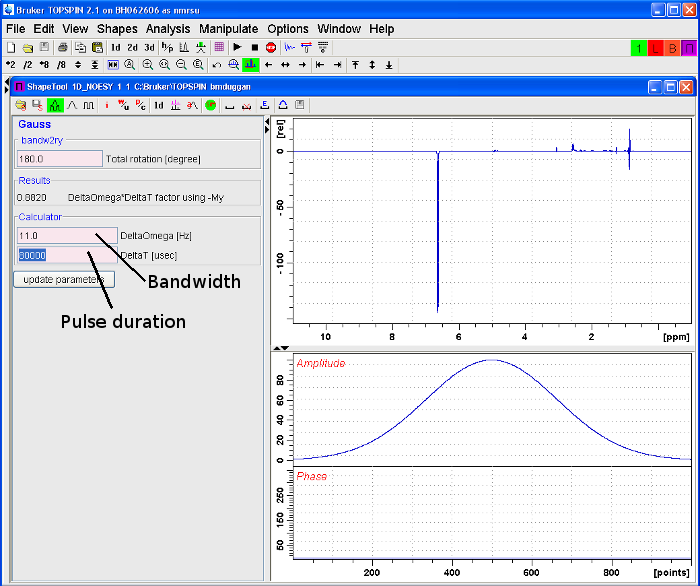
The duration of the selective pulse determines its selectivity. The longer the pulse, the narrower the region that is excited. The region excited by the pulse is known as the bandwidth.

To determine the duration of the pulse first decide on the bandwidth by measuring in Hz the region you wish to excite. Then with Shape Tool open, select "Analysis -> Calculate Bandwidth for ..." from the TopSpin menu bar. The exact option to select depends on the purpose of the shaped pulse. For a 1D selective NOESY, where the shaped pulse is a 180^o pulse refocussing xy magnetisation, the correct option is "Calculate Bandwidth for Refocusing -My". To calculate the duration for a 90^o pulse use "Calculate Bandwidth for Excitation"

After selecting the appropriate bandwidth calculation the pink text boxes in the left panel will change. Check that the value for total rotation is correct. In the bandwidth box labelled "DeltaOmega [Hz]" enter the desired bandwidth and press enter. The value in the pulse duration box, labelled "DeltaT [usec]", will update to give you the pulse duration corresponding to your desired bandwidth. It is also possible to enter a duration and determine the corresponding bandwidth.

Power

The power of the shaped pulse has to be adjusted to match the duration so that enough power is applied to produce the desired rotation of the magnetisation. Different calculation methods are used for classical pulses and for adiabatic ones.

Classical pulses

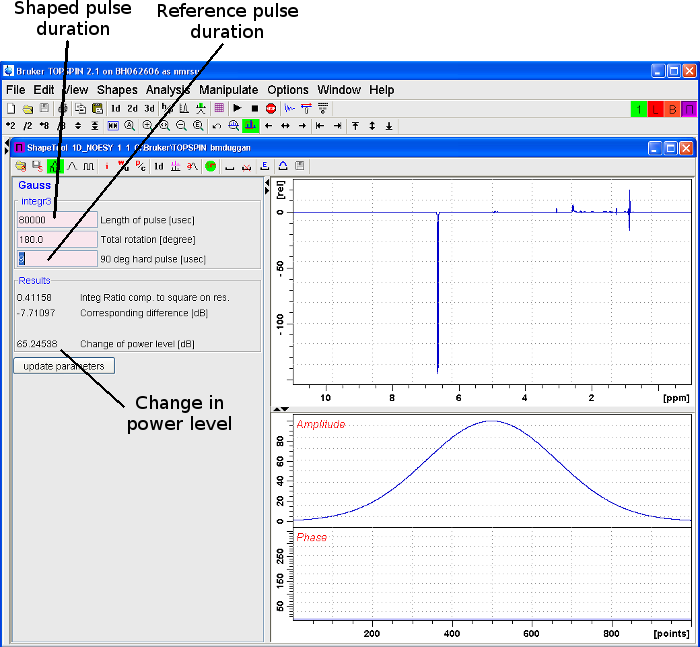
To calculate the power required for a classical shaped pulse select "Analysis -> Integrate Shape" from the TopSpin menubar. The textboxes in the left panel will change. In the topmost textbox enter the duration of the shaped pulse in μsec. Check the total rotation is set correctly. Then, in the third textbox enter the length of the reference pulse, the high power 90^o pulse. This is normally set by the "getprosol" or "gpro" command and can be found by looking up the value of "p1". After setting the three values, pressing enter will update the value labelled "Change of power level [dB]". The change in power level must be added to the power for the reference pulse, i.e. "pl1", and entered as the power for the shaped pulse. For 90^o shaped pulses the power level is normally "sp1", while for 180^o pulses its typically "sp2". Check the pulse sequence comments to be sure.

Adiabatic pulses

For adiabatic pulses (e.g. "Crp60,0.5,20.1", "Crp60_Xfilt.2") the appropriate power calculation option is "Analysis -> Integrate Adiabatic Shape". Selecting this option brings up the same textboxes as for the classical shapes except for the total rotation box. This textbox is missing since the total rotation cannot be adjusted for adiabatic shapes. The power change is calculated by entering the duration of the adiabatic pulse and of the reference pulse, as for the classical shapes.

Written by Brendan Duggan. Last modified 2022-Jul-22

Section 'Sub' Navigation: